Dr. Lalitha Subramanian
About the Author
Aksharam
The absolute value of a real number $x$ is the distance between the graph of $x$ and the origin on the number line.
The absolute value equation $\mid x \mid = 2$ represents the equivalent compound sentence "$x = -2 \quad{or} \quad x=2$". We solve an absolute value equation by first writing it as equivalent disjunction.
Solve $\mid 3x-5\mid =8$
Solution: Writing the equivalent disjunction statements and solving: \begin{array}{rcl} 3x-5 & = & -8 & or & 3x-5 & = & 8\\ 3x & = & -3 & or & 3x & = & 13\\ x & = & -1 & or & x & = & \frac{13}{3}\\ \end{array}
So, the solution set is $\{-1, \frac{13}{3}\}$. Note that the braces enclosing the solution set indicates that the solution set consists of only these numbers.
Solve $\mid 2z-1\mid + 3 = 8$
Solution: First subtract 3 from both sides to form the equation so that the resulting equation has only the absolute value term on the left side. Then form the equivalent disjunction statements and solve: \begin{array}{rcl} \mid 2z-1\mid & = & 5\\ 2z-1 & = & -5 & or & 2z-1 &= & 5\\ 2z &= & -4 & or & 2z & = & 6\\ z & = & -2 & or & z & = & 3\\ \end{array}
So, the solution set is $\{-2, 3\}$.
Solve $4-\mid 3x+1\mid=2$.
Solution: Here, we can rewrite the equation ane solve it as \begin{array}{rcl} \mid 3x+1 \mid -4 & = & -2\\ \mid 3x+1 \mid & = & 2\\ 3x+1 & = & 2 & or & 3x+1=-2\\ 3x & = & 1 & or & 3x & = & -3\\ x & = & \frac{1}{3} & or & x & = & -1\\ \end{array}
So, the solution set is $\{\frac{1}{3}, -1\}$
Absolute value inequalities involve two types of combined inequalities.
While forming the two inequalities, first rewrite the absolute value inequality such that only the absolute value expression is on the left side, and all other numbers/ expressions are on the right side. Then, use the following steps to form the two inequalities:
Step 1: Write the first equation with the expression on the left side without the absolute value, keeping the same inequality and the right side expression.
Step 2: Write the second equation with the same expression on the left side, reversing the inequality, and negative of the right side expression.
Step 3: Solve each equation separately. If the inequality is $\lt$ or $\leq$, the final solution would be the conjunction or the intersection set of these two solution sets. If the inequality is $\gt$ or $\geq$, then the final solution would be the disjunction or the union set of these two solution sets.
Solve the inequality $\mid x + 3 \mid \leq 7$
This problem is a conjunction inequality. So,we form two inequalities with "and" in between. \begin{array}{rcl} x+3 & \leq & 7 & and & x+3 & \geq & -7\\ x & \leq & 4 & and & x & \geq & -10\\ \end{array}
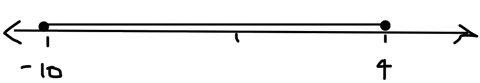
So, the solution set is written in set builder form as $\{x/ -10 \leq x \leq 4\}$, and as an interval $[-10, 4]$. This is shown on the number line as below:
Solve the inequality $\mid 2x-3 \mid \gt 1$.
This problem is a disjunction inequality. So, we form two inequalities with "or" in between \begin{array}{rcl} 2x-3 & \gt & 1 & or & 2x-3 & \lt & -1\\ 2x & \gt & 4 & or & 2x & \lt & 2\\ x & \gt & 2 & or & x &\lt& 1\\ \end{array}
So, the solution set is written in set builder form as $\{x/ x \lt 1 \quad or \quad x \gt 2 \}$, and as an interval $(-\infty, 1) \cup (2, \infty)$. This is shown on the number line as below:

Solve the inequality $\mid x+1\mid \geq \frac{x+4}{2}$.
In this problem, we note that the variable $x$ appears on left as well as right sides. Using the definition of absolute value inequalities, we first split this inequality into two separate inequalities: \begin{array}{rcl} x+1 & \geq & \frac{x+4}{2} & or & x+1 & \leq &-\frac{x+4}{2}\\ 2x+2 & \geq & x+4 & or & 2x+2 & \leq & x+4\\ x & \geq & 2 & or & x &\leq & -2\\ \end{array}
As $x$ appears on both sides, it is advicable that we check both the correctness of the solution set. For thr solution set $x \leq -2$, we substitute a value less than $-2$ on both sides of the inequality: LHS = $|x+1| = |-3+1| = |-2| = 2$; RHS = $\frac{x+4}{2} = \frac{-3+4}{2} = \frac{1}{2}$ ; $2 \geq \frac{1}{2}$. So, the first solution $x \leq -2$ is correct. Similarly, we check the correctness of the second solution by sustituting a value greater than $2$: LHS = $|3+1| = |4| = 4$; RHS = $\frac{3+4}{2} = \frac{7}{2}$. We see that the second solution $x \geq 2$ also works as $4 \geq \frac{7}{2}$. So, the solution set is written in set builder form as $\{x/ x \leq -2 \quad or \quad x \geq 2 \}$, and as an interval $(-\infty, -2) \cup (2, \infty)$.
Solve the following absolute value sentences. For inequality problems, write your solutions as an interval and as inequality.
$\{-6, 0\}$
$(1,4); \quad 1 \lt t \lt 4$
$(-\infty,1] \cup [2, \infty); \quad x \leq 1 \quad or \quad x \geq 2$
$\{-\frac{6}{5}, 2\}$
$(-\infty,1] \cup [5, \infty); \quad x \leq 1 \quad or \quad x \geq 5$
$[2a-4, 2a+4]$; $\quad p \geq 2a-4 \quad or \quad p \leq 2a+4$
$\{-9, -1\}$
$[1.25, 3.75]; \quad 1.25 \lt n \lt 3.75$
$[0,4]; \quad 0 \leq t \leq 4$
$(-\infty, -1) \cup (\frac{1}{3}, \infty); \quad k \lt -1 \quad or \quad k \gt \frac{1}{3}$
$[\frac{3}{2}, 2]; \quad \frac{3}{2} \leq x \leq 2$
$(-\infty, -\frac{7}{2}] \cup [\frac{17}{2}, \infty); \quad t \leq -\frac{7}{2} \quad or \quad t \geq \frac{17}{2}$
No Solution
$(\frac{1}{2}, \frac{5}{2}); \quad \frac{1}{2} \lt x \lt \frac{5}{2} $
$\{1, 4\}$
$(0,2); \quad 0 \lt x \lt 2$
$(-\infty, -\frac{39}{7}) \cup (3, \infty); \quad x \lt -\frac{39}{7} \quad or \quad x \gt 3$
$(1, \frac{5}{3})$; $1 \lt x \lt \frac{5}{3}$
$[-7. \frac{5}{3}]$; $-7 \leq x \leq \frac{5}{3}$
No Solution
$(-\infty, \frac{1}{2}]$; $-\infty \leq x \leq \frac{1}{2}$
No solution.
$[2, 4]$; $2 \leq x \leq 4$
$(-8, 5)$; $-8 \lt x \lt 5$
$(-1, 3)$; $-1 \lt x \lt 3$
$(-\infty, -\frac{7}{4}) \cup (\frac{17}{4}, \infty)$; $x \lt -\frac{7}{4} \quad or \quad x \gt \frac{17}{4}$
No Solution
$\{-6, 36\}$
$(-11, 2)$; $-11 \lt x \lt 2$
$\{2, 7\}$